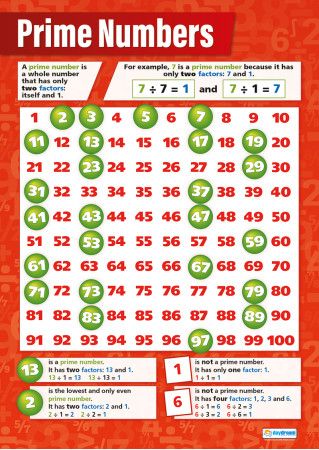
Tuesday, July 14, 2020
Monday, July 6, 2020
Friday, May 29, 2020
NUMBER SYSTEM
NUMBER SYSTEM
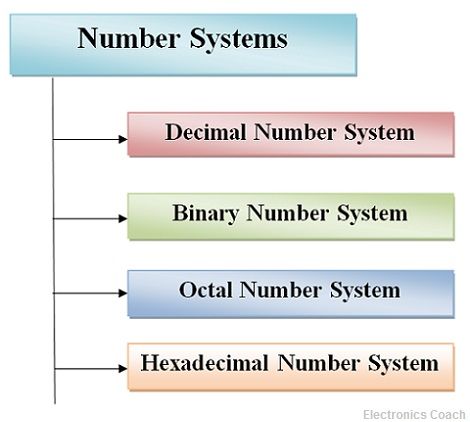
It is the mathematical notation for representing numbers of a given set by using digits or other symbols in a consistent manner.
...
The four most common number system types are:
...
The four most common number system types are:
- Decimal number system (Base- 10)
- Binary number system (Base- 2)
- Octal number system (Base-8)
- Hexadecimal number system (Base- 16)
Binary Numeral System - Base-2
Binary numbers uses only 0 and 1 digits.
B denotes binary prefix.
Examples:
101012 = 10101B = 1×24+0×23+1×22+0×21+1×20 = 16+4+1= 21
101112 = 10111B = 1×24+0×23+1×22+1×21+1×20 = 16+4+2+1= 23
1000112 = 100011B = 1×25+0×24+0×23+0×22+1×21+1×20 =32+2+1= 35
Examples:
278 = 2×81+7×80 = 16+7 = 23
308 = 3×81+0×80 = 24
43078 = 4×83+3×82+0×81+7×80= 2247
Decimal Numeral System - Base-10
Decimal numbers uses digits from 0..9.
These are the regular numbers that we use.
Example:
253810 = 2×103+5×102+3×101+8×100
Examples:
2816 = 28H = 2×161+8×160 = 40
2F16 = 2FH = 2×161+15×160 = 47
BC1216 = BC12H = 11×163+12×162+1×161+2×160= 48146
Numeral systems conversion table
| Decimal Base-10 | Binary Base-2 | Octal Base-8 | Hexadecimal Base-16 |
|---|---|---|---|
| 0 | 0 | 0 | 0 |
| 1 | 1 | 1 | 1 |
| 2 | 10 | 2 | 2 |
| 3 | 11 | 3 | 3 |
| 4 | 100 | 4 | 4 |
| 5 | 101 | 5 | 5 |
| 6 | 110 | 6 | 6 |
| 7 | 111 | 7 | 7 |
| 8 | 1000 | 10 | 8 |
| 9 | 1001 | 11 | 9 |
| 10 | 1010 | 12 | A |
| 11 | 1011 | 13 | B |
| 12 | 1100 | 14 | C |
| 13 | 1101 | 15 | D |
| 14 | 1110 | 16 | E |
| 15 | 1111 | 17 | F |
| 16 | 10000 | 20 | 10 |
| 17 | 10001 | 21 | 11 |
| 18 | 10010 | 22 | 12 |
| 19 | 10011 | 23 | 13 |
| 20 | 10100 | 24 | 14 |
| 21 | 10101 | 25 | 15 |
| 22 | 10110 | 26 | 16 |
| 23 | 10111 | 27 | 17 |
| 24 | 11000 | 30 | 18 |
| 25 | 11001 | 31 | 19 |
| 26 | 11010 | 32 | 1A |
| 27 | 11011 | 33 | 1B |
| 28 | 11100 | 34 | 1C |
| 29 | 11101 | 35 | 1D |
| 30 | 11110 | 36 | 1E |
| 31 | 11111 | 37 | 1F |
| 32 | 100000 | 40 | 20 |
Wednesday, May 6, 2020
Saturday, April 25, 2020
10 VEDIC MATHS TRICKS TO MAKE YOUR CALCULATION FASTER
10 VEDIC MATHS TRICKS TO MAKE YOUR CALCULATION FASTER
Tired of doing calculations
Ever lost marks due to silly mistakes??
Have you spent a looooooooong time calculating answer for a particular problem and still got it wrong.
Don't worry with these tricks your calculation will become easier
How to square a number whose last digit is 5
This vedic maths formula is pretty simple by which you can square any 2-digit number which has 5 in its one’s place.
First, multiply the 1st digit on the left-hand side with 1 and then,
write 25 at the end.
That’s trick 1 for you. You still didn’t get it.
See the example below: –
For example: (75) ² =?
Step 1:75 x 75 = …….25 (at the end)
Step 2:7x (7+1) = 7 x 8 = 56
Therefore, the answer is 5625.
https://youtu.be/cAVph17rWlE
2. Multiplication of a number with 5
Memorizing 5 table is every easy. But as the smaller numbers turn to larger numbers, calculation turns complex.
This vedic maths technique helps you to solve this problem.
Here, you have to take any number and divide it by 2.
If your result is a whole number,
then add 0 (zero digit) at the end of the number.
If it is not a whole number, then add 5 at the end and ignore whatever remainder
you have got.
For example: 8426 x 5 =?
Step 1: 8426 / 2 = 4213
Step 2: It is a whole number, so add 0
The answer will be 8426 x 5 = 42130
Let’s try another: 7337 x 5
Step 1:3773 / 2 = 3668.5
Step 2: This is a fractional number, so we will ignore remainder and add 5 at the end.
The answer will be 7337 x 5 = 36685
3. How to subtract a number from 1000, 10000, 100000 and so on.
This maths technique is very useful when you want accurate subtracted results from 1000, 10000, 100000 and so on……
There is only one formula – Subtract all the digits from 9 and last digit from 10.
For example: 1000 – 876 =?
We simply subtract each figure in 876 from 9 and the last figure from 10.
Step 1. 9 – 8 = 1
Step 2. 9 – 7 = 2
Step 3. 10 – 6 = 4
So, the answer is 1000 – 876 = 124
4. How to Multiply any 2-digit numbers between 11 to 19
This trick is of great help if you are just like me and cannot remember the tables after 10.Once practiced for adequate amount of times, you can calculate the results very fast.
There are in total 4 steps to solve through this type of trick
Step 1: Add the unit digit of smaller number to the larger number.
Step 2: Multiply the sum result by 10.
Step 3: Multiply both number’s unit digit.
Step 4: Add both the numbers (which were involved in step 1 & step 2).
For example: Take 2 numbers like 12 and 15.
Step 1. 15 + 2 =17
Step 2. 17*10 = 170.
Step 3. 2*5 = 10
Step 4. Add the two numbers, 170+10 and the answer is 18
5. Division of a large number by 5
This technique quickly gives the answer
after division of any large number by 5.
There are only two steps which you need to follow.
First, being multiplication of number by 2 and
second being removal of digit after decimal point.
For example: 415 / 5 =?
Step 1. 415 * 2 = 207.5
Step 2. Move the decimal: 207.5 or just 207
6. How to Multiply any two-digit number with 11
Using this mental math trick, multiplication can be done swiftly.
To multiply 45 and 11,
let us imagine that there is a gap between 45
Step 1: Put an imaginary space in between: 45*11= 4_5
Step 2: Just add 4 and 5 and put the result in the imaginary space
So, the answer is: 45 * 11 = 495
Let’s try another number….
36 * 11 = 3 (3+6) 9 = 396
7. Multiplication of any large number by 12
In order to multiply any no. by 12
we need to just double the last digit of the number and then, double each digit and add it to its neighboring number.
For example: 3243 * 12 =?
Let’s break it into simple steps:
Step 1. 3243 * 12 = _____6 (Double of Last Digit 3= 6 )
Step 2. 3243 * 12 = ____16 (Now Double 4= 8, and add it to 3, 8+3=11, 1 will get carry over)
Step 3. 3243 * 12= ___916 (Now Double 2=4, and add it to 4 with carry, 4+4+1=9)
Step 4. 3243* 12= _8916 (Now Double 3=6, and add it to 2, 6+2=8)
Step 5. 3243 * 12= 38916
So your final answer of 3243 * 12 = 38916
8. Multiplication of any 3-digit numbers
Take any two numbers like 204 and 206
Step 1. Now subtract the number at unit place.
204-4=200
206-6=200
Step 2. Now select any no. and add the unit digit of another no.
204+6=210
Step 3. Now multiply, 210×200 = 42000
Step 4. Now multiply the unit digits of both numbers, 4×6=24
Step 5. Add, 42000+24 = 42024
The product of the numbers 204 and 206 is 42024
9. How to calculate square of any number
Calculating squares can be very easy if you are doing it using Vedic maths.
Step 1. Choose a base nearer to the no. whose sq. is to be found.
Step 2. Find the difference of the no. from its base.
Step 3. Add the difference with the no.
Step 4. Multiply the result with the base.
Step 5. Add the product of the sq. of the difference with the result of the above point.
Let’s take an example to understand this: (88) ² =?
Step 1. Choose 100 as base
Step 2. Difference =88-100 = -12
Step 3. Number + difference = 99 + (-12) = 87
Step 4. Multiplying result with base = 87*100 = 8700
Step 5. Adding result with square of difference= 8700 + (-12)² = 8844
10.
Tuesday, April 7, 2020
Wednesday, February 26, 2020
Subscribe to:
Posts (Atom)